Vortex dynamics and wakes
Interactions of plumes with density-stratification
Contacts: Julie Albagnac, Dominique Anne-Archard, Pierre Brancher
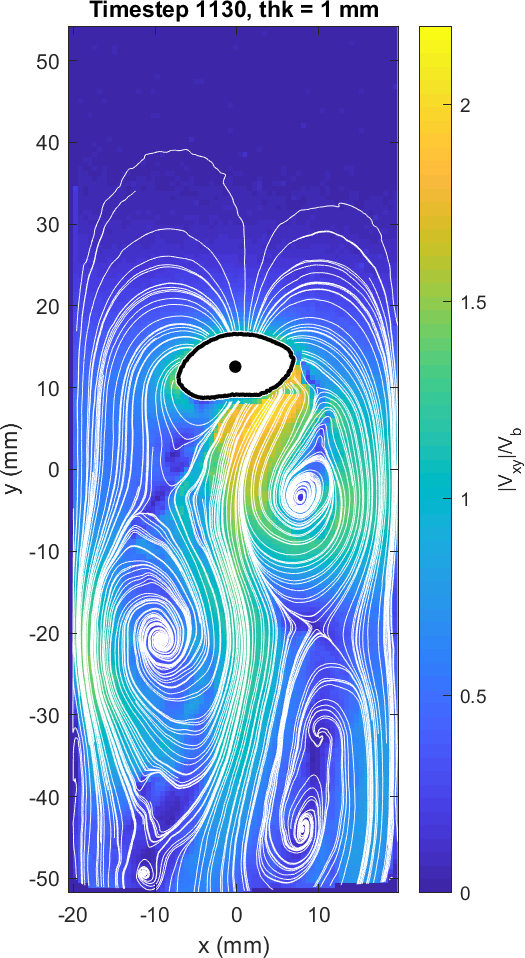
This axis aims at a better understanding of the mixing mechanisms in density stratifications (oceans, atmospheres, industrial devices involving large retention tanks, etc.). For the sake of modeling the mixing, a vortex ring, representative of a plume or a thermal, is propagating in an ambient fluid whose density evolves linearly in the direction of gravity. The control parameters of our experimental device are: the vortex ring intensity, the stratification stiffness and the vortical structure angle of propagation. It is also possible to generate several consecutive rings. Figure shows an illustration of a vortex ring impacting orthogonally the stratification.
Vortex dynamics in non-newtonian viscoelastic fluids
Contacts: Julie Albagnac, Dominique Anne-Archard
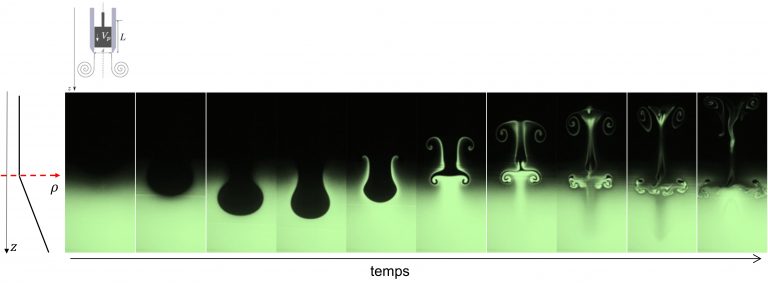
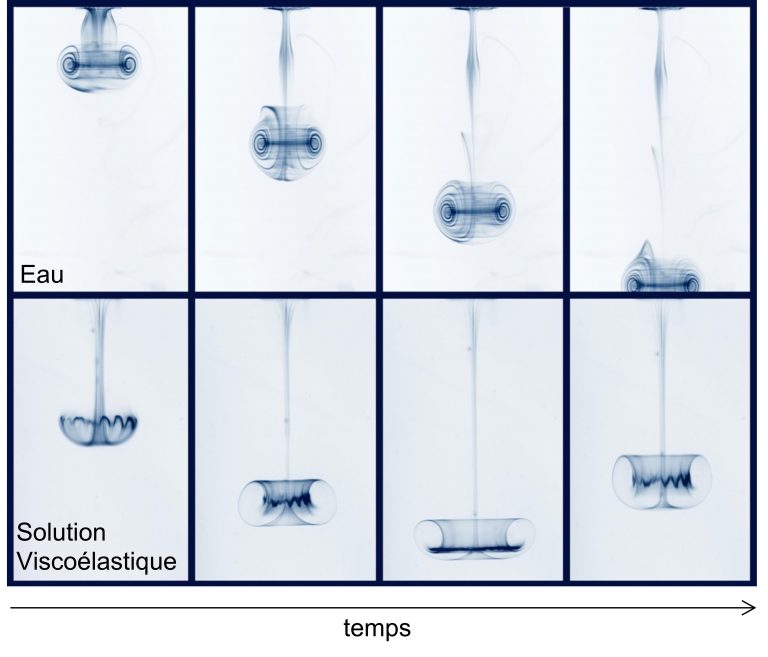
Many flows, both environmental and industrial, involve fluids with original rheology. Some are shear-thinning : their viscosity decreases with shear, or the other way around, shear-thickenning. Some fluids exhibit elastic effects. As examples we can cite lava flows, most of the fluids used in the food industry, granular flows when modeled on a large scale, oil etc. For these flows with particular physical characteristics, transport and mixing mechanisms widely studied for Newtonian fluids (ie, for which the strain is directly proportional to the stress) such as water or air, are no longer valid. In this axis, we address the kinematics of a viscoelastic vortex ring evolving in a fluid initially at rest. An illustration is presented hereafter. In the top row, one can follow a vortex ring propagating in water. On the bottom row, the vortex ring is generated in a tank filled with a viscoelastic solution. The kinematics of the vortex structure in these two configurations is very different. Having a better understanding of the physics of a vortex ring propagating in a non-Newtonian fluid would eventually allow transport or mixing optimization of this kind of solution.
Dynamics of a particle in the vicinity of a vortex
Contacts: Julie Albagnac, Pierre Brancher, Laurent lacaze
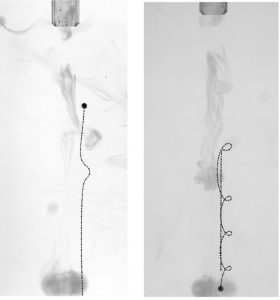
Many flows are heterogeneous, transporting dispersed particles. For instance, one can cite volcanic fumes, clouds, fluids in food or pharmaceutical industry, etc. For all these applications, it is important to describe interactions between a particle, or a set of particles, and the surrounding flow. The objective of this project is to study the weak coupling of a single light inertial particle evolving in the vicinity of a vortex ring, considered as a model vortex structure. The particle trajectory, along with the vortex ring propagation, is followed thanks to 3D visualization.
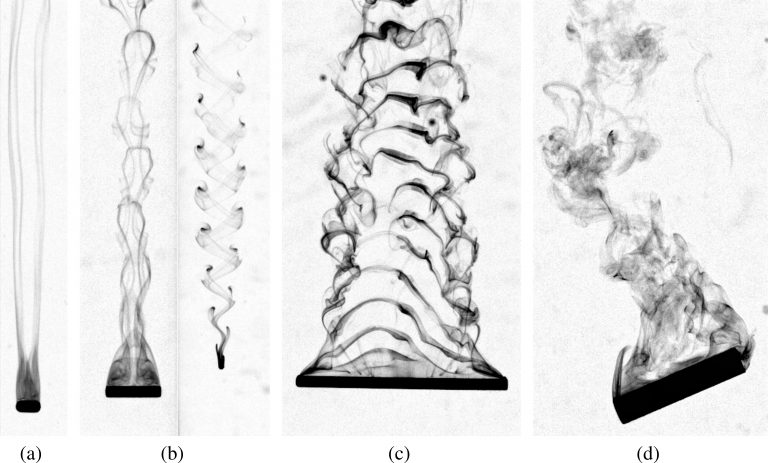
Kinematics and wake of freely falling cylinders at moderate Reynolds numbers
Contacts: Patricia Ern, Véronique Roig
PhD Thesis : Clément Toupoint (2019)
For cylinders in free fall in a liquid at rest in the inertial regime, we explore the diversity of motions and associated wakes over a large range of control parameters (cylinder elongation ratio and volume) in order to find generic scaling laws for the body motion.
Flow induced by a freely rising bubble confined in a thin-gap cell
Contacts: Sébastien Cazin, Véronique Roig,Patricia Ern,
Collaboration: L. Pavlov, M. Cachile and M. V. D’Angelo (Univ. Buenos-aires, Argentina)
We explore the specific complex 3D organization of the motion induced by a bubble freely rising in a thin-gap cell in the inertial regime.